Difference between revisions of "3^4"
(Yet another edit in notation!) |
|||
| Line 12: | Line 12: | ||
To state a set of all possible turns (clicks) of a cell, X- is used. For example: R- includes RU, RF, RD, RB, RA, and RK.<br /> | To state a set of all possible turns (clicks) of a cell, X- is used. For example: R- includes RU, RF, RD, RB, RA, and RK.<br /> | ||
To state a set of all possible turns (clicks) of cells that are joined to the original sticker to be clicked, -X is used. For example, -R includes UR, FR, DR and BR, AR and KR. | To state a set of all possible turns (clicks) of cells that are joined to the original sticker to be clicked, -X is used. For example, -R includes UR, FR, DR and BR, AR and KR. | ||
| − | ===<RK, | + | ===<RK,A->=== |
| − | <RR, | + | <RR,A-> moves mean to only use RK and A- moves. This method is used by Matthew Sheerin. This type of move can replicate 3^3 algorithms on one cell only (i.e. the far cell). An example of this is RK AF RK AF' RK' AF RK' AF'. This is ''the <RK,A-> version'' of R U R' U'.<br /> |
==Sheerin-Zhao Method (Hybrid)== | ==Sheerin-Zhao Method (Hybrid)== | ||
| Line 27: | Line 27: | ||
<li>Cross: Make a cross by solving 8 2C pieces on the far cell.</li> | <li>Cross: Make a cross by solving 8 2C pieces on the far cell.</li> | ||
<li>F2L: Fill in F2L slots by joining F2L pairs (2C/3C) together and inserting them into their respective slots.</li> | <li>F2L: Fill in F2L slots by joining F2L pairs (2C/3C) together and inserting them into their respective slots.</li> | ||
| − | <li>S2L: Fill in S2L slots. This is done by moving a 3C piece and its respective 4C piece onto the farthest cell (i.e. the last layer). Then, OLL algorithms are used to orient the pieces so that they can be joined using < | + | <li>S2L: Fill in S2L slots. This is done by moving a 3C piece and its respective 4C piece onto the farthest cell (i.e. the last layer). Then, OLL algorithms are used to orient the pieces so that they can be joined using <RK,A-> moves. They are then inserted by using <RU or LU, DA> moves.</li> |
| − | <li>OLL: Orient the LL 2C pieces, | + | <li>OLL: Orient the LL 2C pieces, 3C pieces, ''then'' the 4C pieces using OLL-C (corner OLL) algorithms, such as the sune/antisune. Try to get as many corners oriented as possible as well. Setup moves are highly recommended here.</li> |
<li>Pre-PLL: Permute the 2C of the LL using U-perms. </li> | <li>Pre-PLL: Permute the 2C of the LL using U-perms. </li> | ||
| − | <li>PLL: Solve the LL like a 3^3 by using <RK,AF> moves. </li> | + | <li>PLL: Solve the LL like a 3^3 by using <RK,AF> moves. (The cube is rotated at this stage)</li> |
<li>Parity: If the "top face" of the LL is 180 degrees off from the rest of the puzzle, use the <RA,AF> variant of the supercube centers algorithm (R U R' U five times)</li> | <li>Parity: If the "top face" of the LL is 180 degrees off from the rest of the puzzle, use the <RA,AF> variant of the supercube centers algorithm (R U R' U five times)</li> | ||
</ol> | </ol> | ||
| Line 50: | Line 50: | ||
<li>Intuitively align the two pieces so that they lie on the same slice on the far cell.</li> | <li>Intuitively align the two pieces so that they lie on the same slice on the far cell.</li> | ||
<li>Join the pair together and insert the pair into the slot using -U moves.</li> | <li>Join the pair together and insert the pair into the slot using -U moves.</li> | ||
| − | <li>Note: It is possible to insert the slot flipped. There are many shortcuts in forming pairs too. The image below shows the flipped pair.<br /> | + | <li>Repeat 11 more times. <br />Note: It is possible to insert the slot flipped. There are many shortcuts in forming pairs too. The image below shows the flipped pair.<br /> |
[[File:C3a.png|400px]] | [[File:C3a.png|400px]] | ||
</li> | </li> | ||
| Line 62: | Line 62: | ||
<li>Find its respective 4C piece (see F2L for details).<br /> | <li>Find its respective 4C piece (see F2L for details).<br /> | ||
note: try finding pairs that are already on the far cell first.</li> | note: try finding pairs that are already on the far cell first.</li> | ||
| − | <li>If either piece is already inserted and there are no more pairs on the far cell, go down to | + | <li>If either piece is already inserted and there are no more pairs on the far cell, go down to step 6 and use the algorithm to take the piece out of the slot.</li> |
<li>Orient the pieces so that the same colours are on the far cell. Sune and antisune is used. Setup moves can help.</li> | <li>Orient the pieces so that the same colours are on the far cell. Sune and antisune is used. Setup moves can help.</li> | ||
| − | <li>Join the pair together using <RK,A-> moves. | + | <li>Join the pair together using <RK,A-> moves. Turn the far cell until the pair is aligned as shown below:<br /> |
| + | [[File:C4a.png|400px]]<br /> | ||
| + | It is possible for the pair to be "going rightwards" since pairs could come in mirror images. | ||
| + | <li>Insert the piece using <RU,DA> moves or <LU,DA> moves. In the example shown above, since it is left-sided, it can be inserted using: (LU DA LU DA) (LU' DA LU DA'). The next image shows the pair in place.<br /> | ||
| + | [[File:C4b.png|400px]]</li> | ||
| + | <li>Repeat 7 more times.</li> | ||
| + | <li>Here is what it should look like when you are done:<br /> | ||
| + | [[File:C4c.png|400px]]</li> | ||
| + | </ol> | ||
| + | |||
| + | ====OLL==== | ||
| + | <ol> | ||
| + | <li>Use -U variants of OLL algorithms to orient the 2C pieces. (e.g. for line, FU RU AU RU' AU' FU')<br /> | ||
| + | Attempt to orient as many 3C pieces in this step as possible.</li> | ||
| + | <li>If there are any unoriented 3C pieces, orient them using -U variants of corner OLL algorithms such as the sune.<br /> | ||
| + | Note: if there is only one 3c piece left unoriented (see image below), place it so that it's facing you (see f2l slot pic for reference) Use this algorithm if the piece needs to rotate CCW: [AR2 AU' RK2 RU' AR2 AU' LA2 LU']*2. If the piece needs to rotate CW, use [RK2 RU' AR2 AU' LA2 LU' AR2 AU']*2.<br /> | ||
| + | [[File:C5a.png|400px]] | ||
| + | </ol> | ||
Revision as of 12:35, 6 August 2012
Contents
[hide]Roice Method
This method is the 4D version of the "ultimate solution for the rubik's cube's.
check it out here: "The Ultimate Solution to the 3^4"
Notation
The notation is similar to that of the 3^3. There are 8 cells, six of them using the same letters as that in the 3^3: U (up), D (down), F (front), B (back), R (right), L (left). The "near" and "far" cells are named A (ana) and K (kata), respectively.
Each cell in turn uses WCA Official Notation (standard notation).
The first letter determines the cell to click on. The second letter determines the sticker on the cell (has to be 2C piece) to click on For example:
RK means to click the sticker on the right cell that joins to the near cell.
AF means to click the sticker on the far cell that joins to the front cell.
FA means to click the sticker on the front cell that joins to the far cell.
To state a set of all possible turns (clicks) of a cell, X- is used. For example: R- includes RU, RF, RD, RB, RA, and RK.
To state a set of all possible turns (clicks) of cells that are joined to the original sticker to be clicked, -X is used. For example, -R includes UR, FR, DR and BR, AR and KR.
<RK,A->
<RR,A-> moves mean to only use RK and A- moves. This method is used by Matthew Sheerin. This type of move can replicate 3^3 algorithms on one cell only (i.e. the far cell). An example of this is RK AF RK AF' RK' AF RK' AF'. This is the <RK,A-> version of R U R' U'.
Sheerin-Zhao Method (Hybrid)
This method is an attempt to make a 4D analogue of the Friedrich, or CFOP method. Using this method results in around 650 to 900 moves, even if no shortcut turns are used. This method needs significant improvement because of the move count required for the last layer.
Prerequisites
- Knowledge of how the cube rotates.
- The Friedrich Method (F2L/OLL/PLL). 2-look OLL/PLL is enough.
Summary of the Method
- Cross: Make a cross by solving 8 2C pieces on the far cell.
- F2L: Fill in F2L slots by joining F2L pairs (2C/3C) together and inserting them into their respective slots.
- S2L: Fill in S2L slots. This is done by moving a 3C piece and its respective 4C piece onto the farthest cell (i.e. the last layer). Then, OLL algorithms are used to orient the pieces so that they can be joined using <RK,A-> moves. They are then inserted by using <RU or LU, DA> moves.
- OLL: Orient the LL 2C pieces, 3C pieces, then the 4C pieces using OLL-C (corner OLL) algorithms, such as the sune/antisune. Try to get as many corners oriented as possible as well. Setup moves are highly recommended here.
- Pre-PLL: Permute the 2C of the LL using U-perms.
- PLL: Solve the LL like a 3^3 by using <RK,AF> moves. (The cube is rotated at this stage)
- Parity: If the "top face" of the LL is 180 degrees off from the rest of the puzzle, use the <RA,AF> variant of the supercube centers algorithm (R U R' U five times)
Method
Cross
- Pick the colour that you will be using for the near cell (the cell not inside the viewpoint). In this example, cyan is chosen.
- Rotate the cube so that the cell with that specified colour is now the far cell.
- Twist the puzzle so that all 2C (face) pieces connecting to the near cell are oriented and permuted correctly.
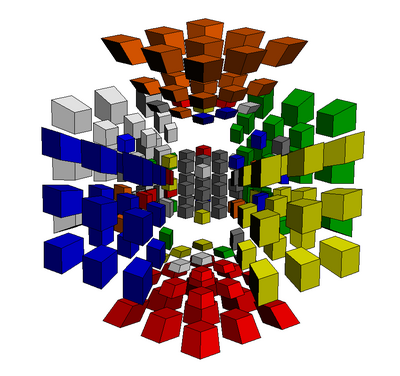
This image shows the solved cross.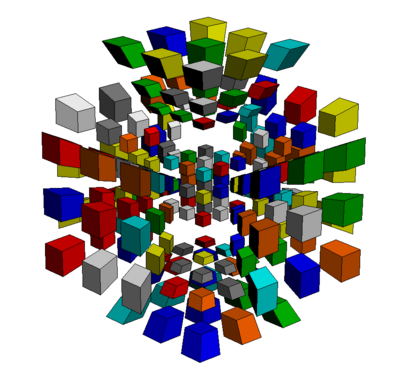
F2L
- Find any 2C piece that does not include a sticker with the colour of the far cell (i.e. grey).
- Find the 3C piece with the stickers the same colour as that of the 2C piece's and of the near cell. For example, if the 2C piece's colours were red and yellow and the near cell piece was cyan, the 3C piece would be red-yellow-cyan.
- Intuitively align the two pieces so that they lie on the same slice on the far cell.
- Join the pair together and insert the pair into the slot using -U moves.
- Repeat 11 more times.
Note: It is possible to insert the slot flipped. There are many shortcuts in forming pairs too. The image below shows the flipped pair.
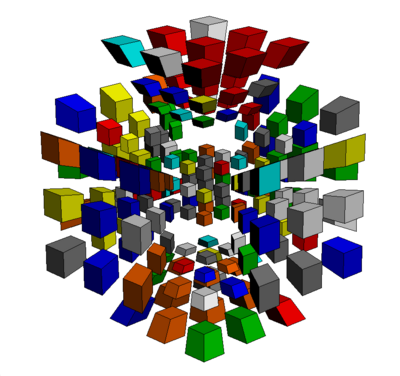
- Here is what it should look like when you are done:
S2L
- Find any 3C piece that does not include a sticker with the colour of the far cell (i.e. grey).
- Find its respective 4C piece (see F2L for details).
note: try finding pairs that are already on the far cell first. - If either piece is already inserted and there are no more pairs on the far cell, go down to step 6 and use the algorithm to take the piece out of the slot.
- Orient the pieces so that the same colours are on the far cell. Sune and antisune is used. Setup moves can help.
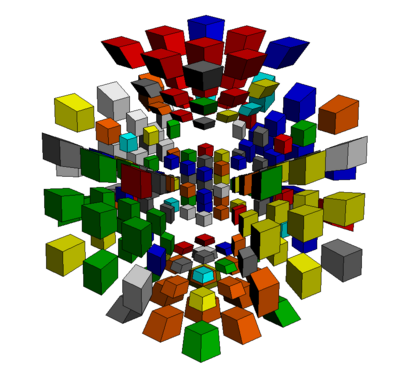
- Join the pair together using <RK,A-> moves. Turn the far cell until the pair is aligned as shown below:

It is possible for the pair to be "going rightwards" since pairs could come in mirror images. - Insert the piece using <RU,DA> moves or <LU,DA> moves. In the example shown above, since it is left-sided, it can be inserted using: (LU DA LU DA) (LU' DA LU DA'). The next image shows the pair in place.
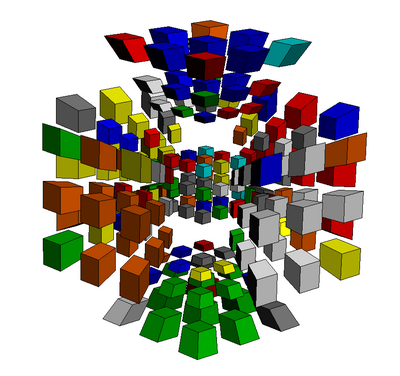
- Repeat 7 more times.
- Here is what it should look like when you are done:
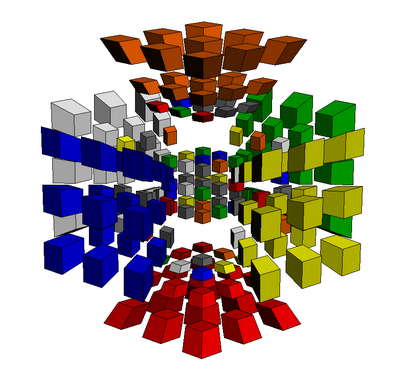
OLL
- Use -U variants of OLL algorithms to orient the 2C pieces. (e.g. for line, FU RU AU RU' AU' FU')
Attempt to orient as many 3C pieces in this step as possible. - If there are any unoriented 3C pieces, orient them using -U variants of corner OLL algorithms such as the sune.
Note: if there is only one 3c piece left unoriented (see image below), place it so that it's facing you (see f2l slot pic for reference) Use this algorithm if the piece needs to rotate CCW: [AR2 AU' RK2 RU' AR2 AU' LA2 LU']*2. If the piece needs to rotate CW, use [RK2 RU' AR2 AU' LA2 LU' AR2 AU']*2.